Euler and the zeta function

Leonhard Euler (1707-1783)

Zeta values calculated by Euler

I have misunderstood about the values of the Riemann zeta function which Euler had calculated. It was because I had not read his original papers. The reflection made me make this page in order not to make such a mistake any more.

Euler calculated six special values of the zeta function in the paper E41 (1734/35), seven more values (the total is 13) in the textbook E101 (1745), and four more values (the total is 17) in the paper E352 (1749). It should be observed that he stopped calculating at 2k=12 (resp. 2k=34=37-3) in E41 (resp. E352). Of all irregular pairs (p,2k), the smallest index is 2k=12, and the smallest prime number is p=37. If you have a proof which shows that Euler realized about these facts, please let me know. (June, November, 2004)
A proof

A reason of "beau" in the title of E352

I had another question: Why did Euler use 'beau' for the title of E352? We usually avoid using such emotional words in papers.

There was a hint in the first page of the paper. Euler expressed the positive values of the zeta function as the moon, and the negative values of the function as the sun. The moon and the sun... I realized that the functional equation is a "solar eclipse"! That is, the moon (quiet positive zeta values) tries to hide the sun (violent negative zeta values), however the remaining part (giant Gamma function) (harmonic cosine function) / (mysterious powers of the circular constant) is still shining! I really felt the beauty as Euler might have. In the following graph, the logarithm value of |zeta(s)| of the sun (resp. moon) is the left-hand(resp. right-hand) side. As the zeta is an alternative sum, there is no pole.

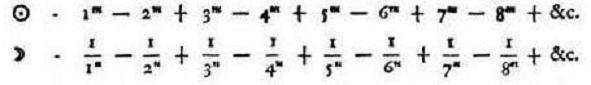
At that time, I didn't know whether Euler had observed a beautiful solar eclipse. So I searched websites and knew that he had observed the solar eclipse on July 25, 1748 (related papers E117, E142). Moreover it was really an annular eclipse!

1748 Jul 25 11:27 A 122 0.518 0.946 48.7N 24.6E 59 231 05m12s
FIVE MILLENNIUM CATALOG OF SOLAR ECLIPSES 1701-1800
(Berlin 52.52N 13.40E) (Homann and Doppelmayr Astronomy Print)

[The path of the annular eclipse in 1748]

Let's imagine Euler's feeling by the following picture.

[Functional equation of Riemann zeta function]

(November, 2004)

Euler left St Petersburg on 19 June 1741,arriving in Berlin on 25 July 1741 (37 days).
It was just 7 years before the annular eclipse.
(October, 2006)

E41 -- De summis serierum reciprocarum (On the sums of series of reciprocals)
[written 1735, presented 1734, published 1740.] Paper 1 Special values 1

E72 -- Variae observationes circa series infinitas (Various observations about infinite series)
[written 1737, presented 1737, published 1744.] Paper 2 Euler product 1 Euler product 2

E101 -- Introductio in analysin infinitorum, volume 1 (Introduction to the Analysis of the Infinite, volume 1)
[written 1745, presented 1748, published 1748.] Textbook Special values 2 Approximated values of the special values 1 Approximated values of the special values 2

E352 -- Remarques sur un beau rapport entre les séries des puissances tant directes que réciproques (Remarks on a beautiful relationship between series of powers and reciprocals of powers)
[written 1749, presented 1761, published 1768.] Paper 3 Special values 3 Special values 4 Functional equation 1 Functional equation 2 Functional equation 3

E477 -- Meditationes circa singulare serierum genus
[written 1771, presented 1775, published 1776.] Paper 4 Double zeta value

Thanks to Prof. N. Kurokawa and Prof. H. Furusho.

Reg: 2005, Feb 19
Title: Euler and the zeta function
Method:w ebpage
Content:
https://math0.pm.tokushima-u.ac.jp/~hiroki/major/euler-e.html
EP: 34301

Index